Research at the Institute of Mechanics and Computational Mechanics

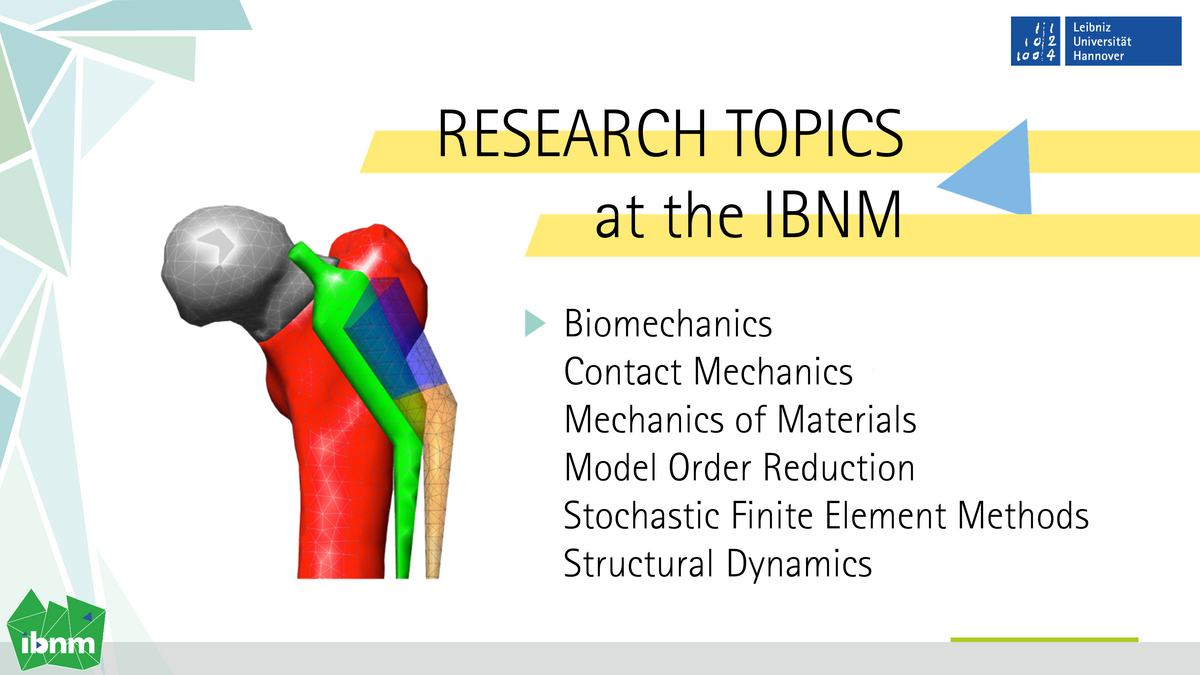
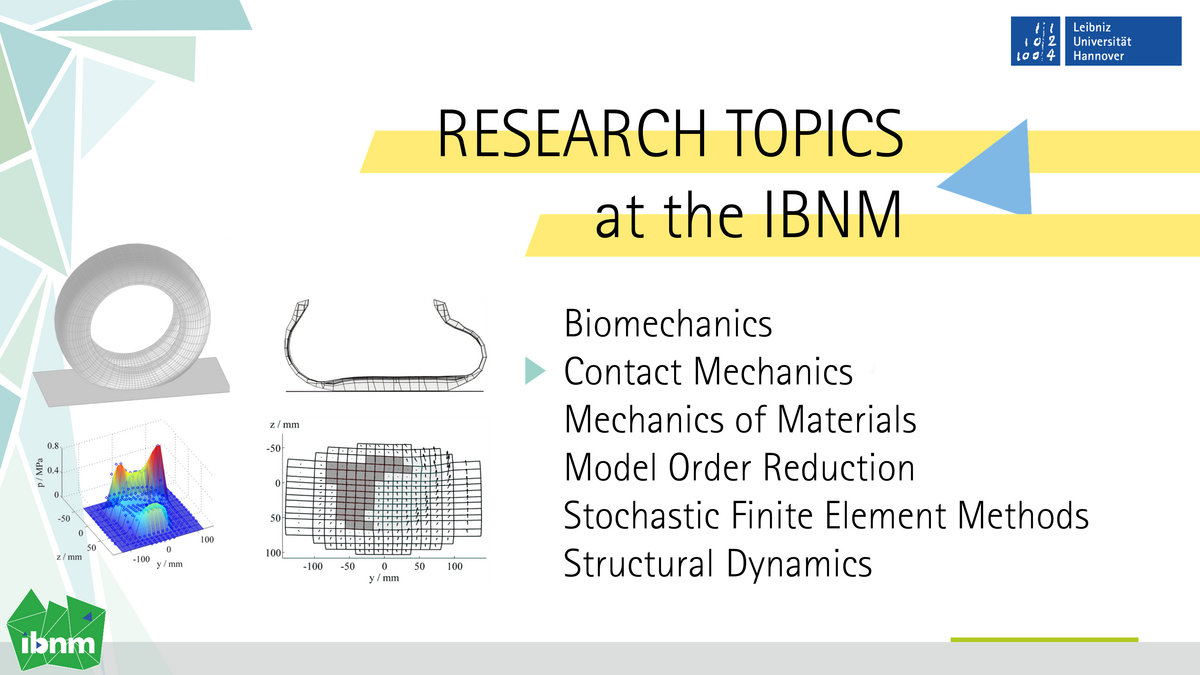
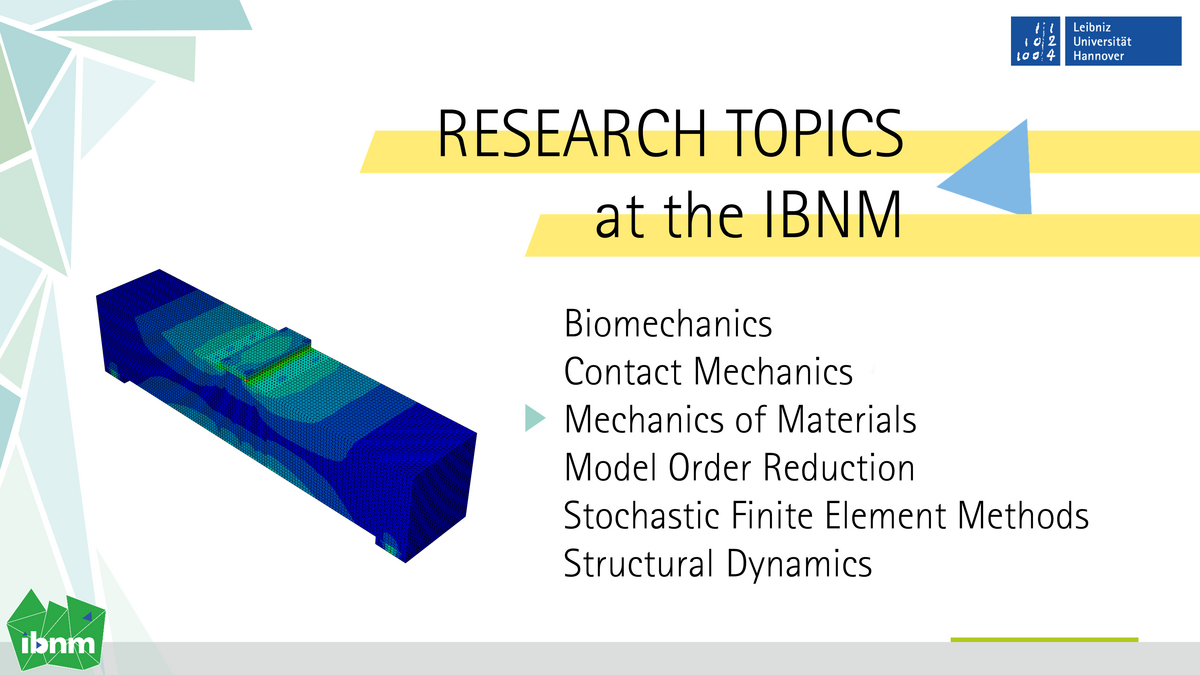
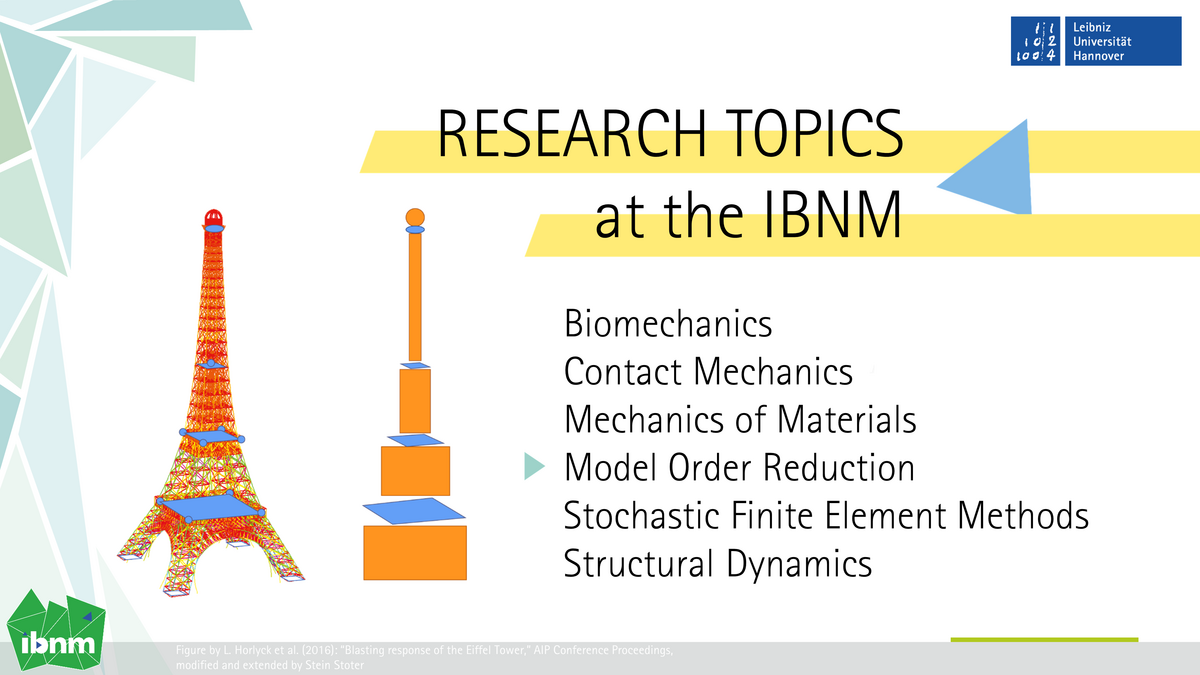
Our research expertise is in the fields of modelling, mathematical description and the development of related computational techniques for sophisticated problems in continuum mechanics. This covers problems such as:

• Constitutive description of the inelastic properties of materials and numerical algorithms for robust computations

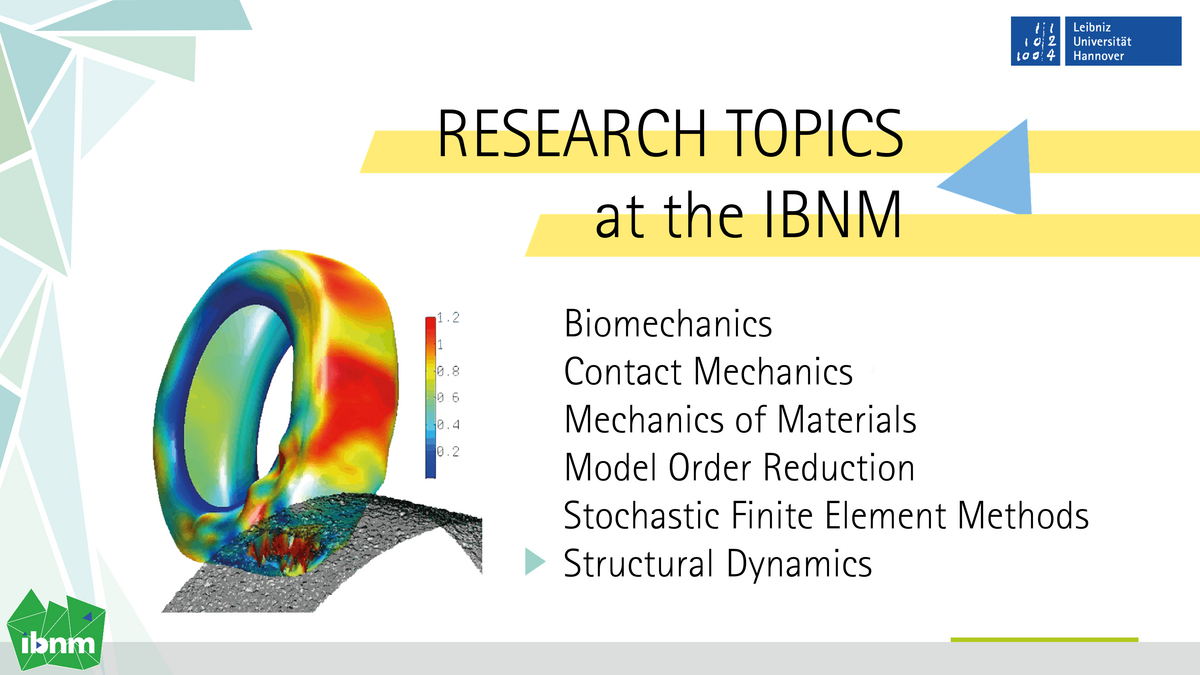
• Efficient and reliable algorithms for (rolling-) contact problems

• Sophisticated computational techniques for time dependent problems, like structural dynamics, damage and fatigue, aging and growth

• Modelling approaches for multi-scale and multi-physics problems

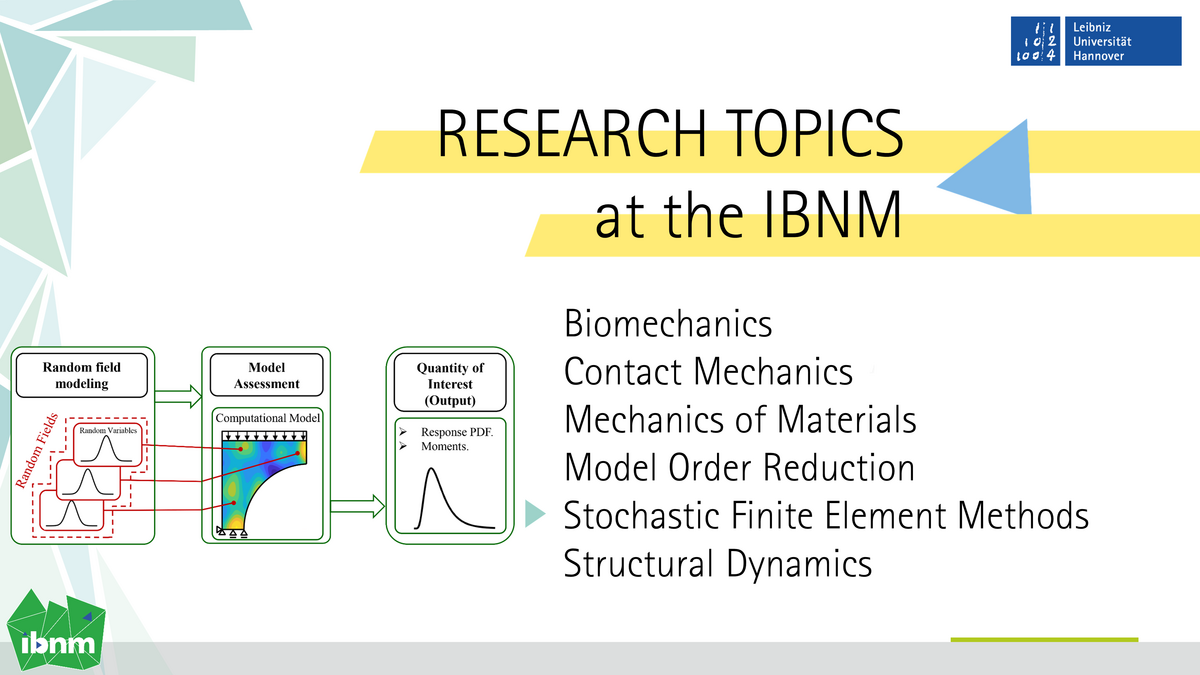
• Computational methods for uncertainty treatment

We are researching sophisticated numerical methods in the fields:

• Arbitrary Lagrangian Eulerian (ALE) computational techniques

• Time Discontinuous Galerkin (TDG) for Advection-Diffusion-Reaction (ADR-) equations

• Discontinuous Galerkin (DG) methods for high dimensional problems

• Adaptive schemes for consistent Molecular-Dynamics (MD) - Finite Element (FE) coupling

• Stochastic Finite Element Methods (SFEM)

• Model reduction techniques